Wave equation for motionless skeleton models

Kirchhoff first developed a theory of sound propagation in tubes with circular cross-section accounting for both viscosity and thermal conductivity of the air. Zwikker & Kosten restricted this theory to a narrow frequency range and a narrow radius range but ensuring that visco-inertial and thermal effects can be calculated separately (using a complex mass density and a complex bulk modulus) without noticeable deviations from Kirchhoff's theory. These mass density and complex bulk modulus functions are denoted as $\widetilde{\rho}_{eq}$ and $\widetilde{K}_{eq}$ respectively hereafter.

From Zwikker & Kosten work the wave equation for the acoustic pressure wave inside a tube is:

This equation is analogous to the Helmholtz equation used to described the sound propagation in free air (without any dissipation). However, for porous media, the mass density $\widetilde{\rho}_{eq}$ and the bulk modulus $\widetilde{K}_{eq}$ are complex functions of the frequency and of the pore shape.

Motionless skeleton models aim at providing expressions of $\widetilde{\rho}_{eq}$ and $\widetilde{K}_{eq}$ for the acoustics frequency spectrum and for given pore shapes.

Obviously, the wave equation for motionless skeleton materials can be recovered from Biot's theory. For such a material, the displacement vector $\underline{u}$ of the skeleton and its strain tensor $\underline{\underline{\varepsilon}}$ are equal to zero. Under these displacements and strains conditions, the system of equations describing the motion of a porous material reduces to one equation (i.e. only one compressional wave: $P_{2}$, can propagate in the fluid phase):

This last equation is equivalent to the first reported one.

Overview of the different motionless skeleton models

Three classes of models (i.e. expressions of $\widetilde{\rho}$ and $\widetilde{K}$ as functions of the frequency and of the pore shape) can be listed.
Empirical models which usually require to know a small number of parameters (or information). They are very popular and still very used in spite of their restrictive limits.
Analytical models are valid for porous materials with simple pore morphologies e.g. slit-like pores or parallel cylindrical pores with a singular cross-section (circular, square, triangular) see figure below.
Finally, semi-phenomenological models have been developed for more complicated pore morphologies. For these latter models, only the asymptotic behaviors are known. A behavior between these asymptotes is assumed without it has been mathematically proven.

Linking micro-structure to macro-properties

This research field is an attempt to predict the acoustical macro-properties or performances of a porous material (e.g. sound transmission loss of a given material sample) from the knowledge of its micro-structure (e.g. pore size for foam or fiber size for fibrous...) with or without a prior computation of the acoustic parameters introduced above. Read more on this topic.

References

The references used in this page are reported hereafter.

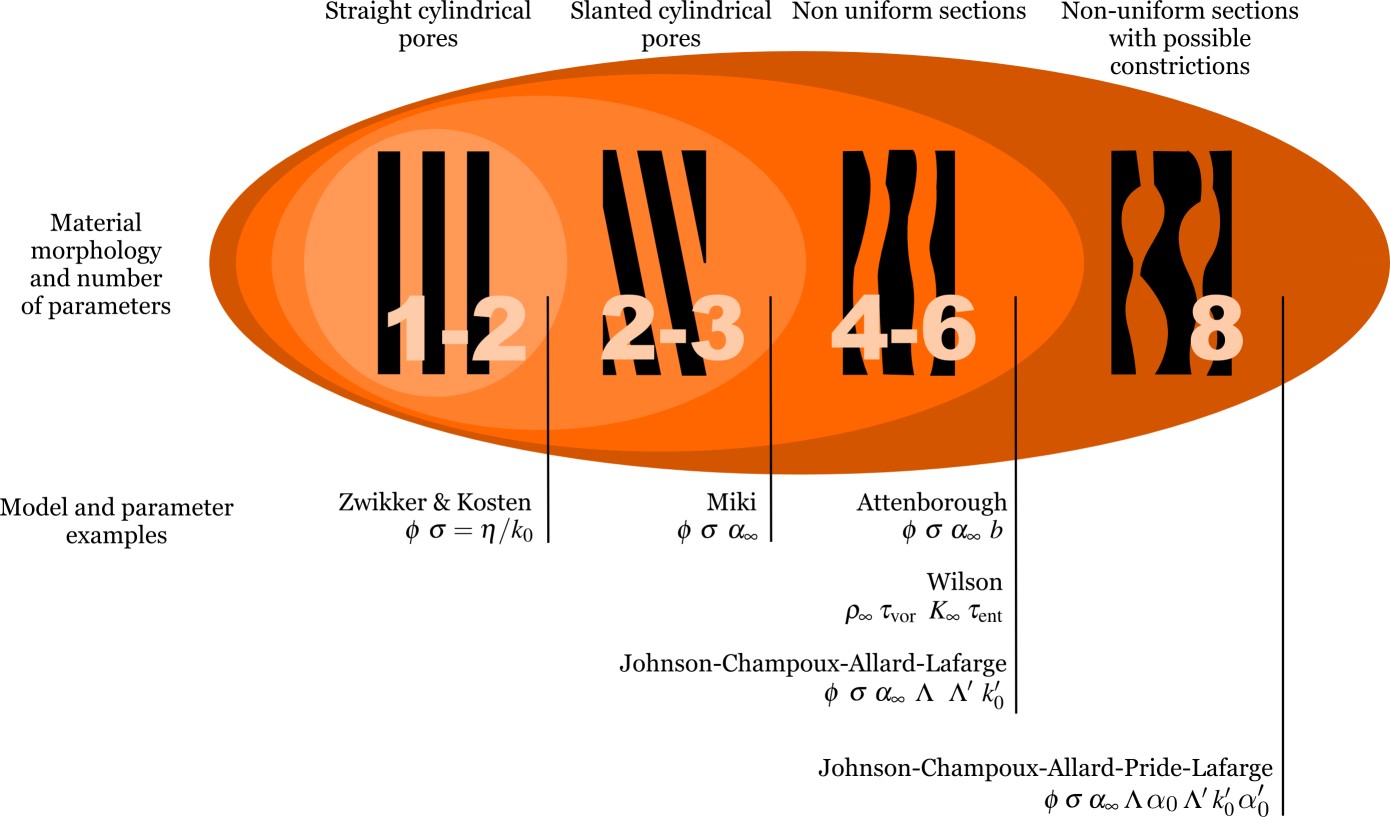
[ZK49] Zwikker C. and Kosten C. W., Sound absorbing materials, Elsevier, New-York, 1949.

[Att83] Attenborough K. Acoustical characteristics of rigid fibrous absorbents and granular materials, J. Acoust. Soc. Am. 73(3), pp.785–799, 1983.

[Sti91] Stinson M. R., The propagation of plane sound waves in narrow and wide circular tubes, and generalization to uniform tubes of arbitrary cross-sectional shape, J. Acoust. Soc. Am. 89(2), 1991, pp. 550-558 ■

[DB70] Delany M. E. and Bazley E. N., Acoustical properties of fibrous absorbent materials, Applied Acoustics 3, 1970, pp. 105-116 ■

[Mik90a] Miki Y., Acoustical properties of porous materials - Modifications of Delany-Bazley models, J. Acoust. Soc. Jpn (E). 11(1), 1990, pp. 19-24 ■

[Mik90b] Miki Y., Acoustical properties of porous materials - Generalizations of empirical models, J. Acoust. Soc. Jpn (E). 11(1), 1990, pp. 25-28 ■

[HGD16] K. V. Horoshenkov, J.-P. Groby, O. Dazel,Asymptotic limits of some models for sound propagation in porous media and the assignment of the pore characteristic lengths, J. Acoust. Soc. Am. 139 (5), 2016, pp. 2463–2474.

[HHG19] K. V. Horoshenkov, A. Hurrell, J.-P. Groby, A three-parameter analytical model for the acoustical properties of porous media J. Acoust. Soc. Am. 145 (4), 2019, pp. 2512–2517.

  [Wil93] Wilson D. K., Relaxation-matched modeling of propagation through porous media, including fractal pore structure, J. Acoust. Soc. Am. 94(2), 1993, pp. 1136-1145 ■

[JKD87] Johnson D. L., Koplik J. and Dashen R., Theory of dynamic permeability and tortuosity in fluid-saturated porous media, J. Fluid Mech. 176, 1987, pp. 379-402 ■

[CA91] Champoux Y. and Allard J.-F., Dynamic tortuosity and bulk modulus in air-saturated porous media, J. Appl. Phys. 70, 1991, pp. 1975-1979 ■

[LLAT97] Lafarge D., Lemarinier P., Allard J.-F. and Tarnow V., Dynamic compressibility of air in porous structures at audible frequencies, J. Acoust. Soc. Am. 102(4), 1997, pp. 1995-2006 ■